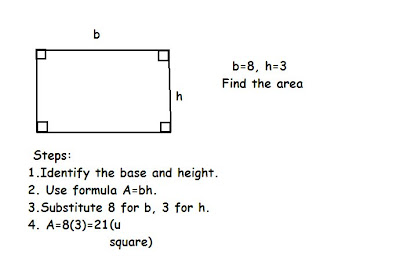
Tuesday, January 27, 2009
Wednesday, January 21, 2009
Thursday, January 15, 2009
6.3 Proving Quadrilaterals are Parallelograms
In this section, we learned how to prove quadrilaterals are parallelograms.
Here are some problems you might find from this section and how to do them.
Firstly, you might be asked to write a proof to prove a quadrilateral is a parallelogram.
Example 1

Given:Angle D is supplementary to angle A and angle C
Prove ABCD is a parallelogram.
1) Angle D is supplementary to angle A and angle C
2) Line AB is parallel to line DC
3) Line AD is parallel to line BC
4) ABCD is a parallelogram
Reasons
1) Given
2) Consecutive Interior Angles Converse
3) Consecutive Interior Angles Converse
4) Definition of a parallelogram
Example 2
You might be asked to decide if there's enough information to prove a quadrilateral is a parallelogram.

If opposite sides of a quadrilateral are congruent, then it's a parallelogram.
Here are some problems to try on your own.
Are you given enough info to decide if the quadrilateral is a parallelogram?
1)

2)

3)

4)

Given: Angle E is congruent to angle G
Angle F is congruent to angle H
Prove: EFGH is a parallelogram
Directions: Use the definition or theorem to prove that HIJK is a parallelogram.
H(-1,6), I(3,5), J(5, -3), K(1,-2).
5) Definition of a parallelogram
Here are some problems you might find from this section and how to do them.
Firstly, you might be asked to write a proof to prove a quadrilateral is a parallelogram.
Example 1

Given:
Prove ABCD is a parallelogram.
1) Angle D is supplementary to angle A and angle C
2) Line AB is parallel to line DC
3) Line AD is parallel to line BC
4) ABCD is a parallelogram
Reasons
1) Given
2) Consecutive Interior Angles Converse
3) Consecutive Interior Angles Converse
4) Definition of a parallelogram
You might be asked to decide if there's enough information to prove a quadrilateral is a parallelogram.

If opposite sides of a quadrilateral are congruent, then it's a parallelogram.
Here are some problems to try on your own.
Are you given enough info to decide if the quadrilateral is a parallelogram?
1)

2)

3)

4)

Given: Angle E is congruent to angle G
Angle F is congruent to angle H
Prove: EFGH is a parallelogram
Directions: Use the definition or theorem to prove that HIJK is a parallelogram.
H(-1,6), I(3,5), J(5, -3), K(1,-2).
5) Definition of a parallelogram
6.4 Rhumbuses, Rectangles, and Squares
6.4 Rhombuses, Rectangles, and Squares
In section 6.4 you will learn about three special types of parallelograms the Rhombus, Rectangle, and the Square. This section is very interesting and fun to study. There are breath taking corollaries and theorems in this section that will help you understand this section a whole lot easier.
The Rhombus:
A Rhombus is a parallelogram with four congruent sides. A
Rhombus Corollary is a quadralateral only if it has four
congruent sides.
The Rectangle:
A Rectangle has four congruent sides. A Rectangle Corollary is
a quadralateral that is a rectangle only if it has four right angles.

The Square:
A Square is a shape with four right angles and four congruent
sides. A Square Corollary is a quadrilateral that is a square only
if it is a rhombus and a rectangle.
Notes/Tips:
* When solving problems that have to do with a rhombues set all sides equal to each other.
*Use properties of sides and angles of Rhombuses, Rectangles, and Squares.
*Theorems will help you solve the equations alot faster.
*Use Properties of Diagnols of Rhombuses, Rectangles and Squares.
Questions:
What information do you need to figure out that this is a Square?
Distance of AB=5x+10
Distance of BC=10x
 Find the value of x. (Use the tips for help)
Find the value of x. (Use the tips for help)
Distance of AD=2y+3
Distance of DC=5y-6
O=65 degrees

Solve for y.(some information is less than they appear)
Consider rectangle JKLM shown in the figure.
If JL = 6y - 21 and MN = 2y + 9, find y.
 Hint: The diagnols are congruent.
Hint: The diagnols are congruent.
 With all the information in the picture, can you tell if all sides of the square are congruent? Explain.
With all the information in the picture, can you tell if all sides of the square are congruent? Explain.
In section 6.4 you will learn about three special types of parallelograms the Rhombus, Rectangle, and the Square. This section is very interesting and fun to study. There are breath taking corollaries and theorems in this section that will help you understand this section a whole lot easier.
The Rhombus:
A Rhombus is a parallelogram with four congruent sides. A
Rhombus Corollary is a quadralateral only if it has four
congruent sides.

The Rectangle:
A Rectangle has four congruent sides. A Rectangle Corollary is
a quadralateral that is a rectangle only if it has four right angles.

The Square:
A Square is a shape with four right angles and four congruent
sides. A Square Corollary is a quadrilateral that is a square only
if it is a rhombus and a rectangle.
Notes/Tips:
* When solving problems that have to do with a rhombues set all sides equal to each other.
*Use properties of sides and angles of Rhombuses, Rectangles, and Squares.
*Theorems will help you solve the equations alot faster.
*Use Properties of Diagnols of Rhombuses, Rectangles and Squares.
Questions:
What information do you need to figure out that this is a Square?

Distance of AB=5x+10
Distance of BC=10x
 Find the value of x. (Use the tips for help)
Find the value of x. (Use the tips for help)Distance of AD=2y+3
Distance of DC=5y-6
O=65 degrees

Solve for y.(some information is less than they appear)
Consider rectangle JKLM shown in the figure.
If JL = 6y - 21 and MN = 2y + 9, find y.
 Hint: The diagnols are congruent.
Hint: The diagnols are congruent. With all the information in the picture, can you tell if all sides of the square are congruent? Explain.
With all the information in the picture, can you tell if all sides of the square are congruent? Explain.
Wednesday, January 14, 2009
6.7: Areas of Triangles and Quadrilaterals
These are problems that you should do to test how much you understand ( just do these problems when you finish reading the tips for remembering the martirals )
Practice Problems

In this section 6.7, you will learn how to find the areas of Triangles and Quadrilaterals.
This section contains 3 parts: examples, tips and problems. All these are instructed in the simplest way so that you can understand the lesson easily. And you'll be suprised that it's easier than what you thought!
First, follow these examples.
Examples
1) Area of a Parallelogram

2) Area of a Triangle


Ok, I'm sure that now you absolutely understand how to use the formulas. Besides, remembering these formulas is also very important. But for somebody, it'll be very hard and spend a lot of time remembering them so these are the useful tips that can make you be a super memorized-Maths student.
Practice Problems

In this section 6.7, you will learn how to find the areas of Triangles and Quadrilaterals.
This section contains 3 parts: examples, tips and problems. All these are instructed in the simplest way so that you can understand the lesson easily. And you'll be suprised that it's easier than what you thought!
First, follow these examples.
Examples
1) Area of a Parallelogram

2) Area of a Triangle

3) Area of a Trapezoid
4) Area of a Kite ( the same as area of a Rhombus )

Ok, I'm sure that now you absolutely understand how to use the formulas. Besides, remembering these formulas is also very important. But for somebody, it'll be very hard and spend a lot of time remembering them so these are the useful tips that can make you be a super memorized-Maths student.
How to better understand the materials?
Do homework ( if you have more times, do more extra problems) for each lesson you have learnt.
If you have troubles with problems, think ..think before asking for help. This way will make you a better thinker in the long run.
Review the mistakes in our quizzes, tests, homework or any problems in our class so that we won’t make any same mistakes.
How to remember the formulas?
The best way to remember is to keep on saying the formulas while you are writing down the formulas.
You can remember them by writing down the formulas as much as you can without looking at.
You can write the formulas on notes with bright color and paste it on everywhere that you often see them.
Do homework ( if you have more times, do more extra problems) for each lesson you have learnt.
If you have troubles with problems, think ..think before asking for help. This way will make you a better thinker in the long run.
Review the mistakes in our quizzes, tests, homework or any problems in our class so that we won’t make any same mistakes.
How to remember the formulas?
The best way to remember is to keep on saying the formulas while you are writing down the formulas.
You can remember them by writing down the formulas as much as you can without looking at.
You can write the formulas on notes with bright color and paste it on everywhere that you often see them.
Practice problems ( they're on the top, there're some problems so we have to move the "Practice Problems" to the top of the section.Sorry)
Tuesday, January 13, 2009
6.5 Trapezoids and Kites
Trapezoids and Kites: Example Problems
-Decide which one best describes a trapezoid.
-Decide which one best describes a trapezoid.
a) a shape with one pair of parallel sides, and four sides overall
b) has no parallel sides
a) three sides are congruent to each other.
-Decide which one is a trapezoid
6.1 Polygon
In this section you've learned how to identify the different polygons and how to find the measures of interior angles of a quadrilateral. Here are some examples:
A. Tell whether the figure is a polygon.
1.

Solution: Yes, it is a polygon because it's a closed figure that has 3 or more sides connecting at the vertices.
2.

Solution: No, it is not a polygon because it is not a closed figure.
B. Tell whether the polygon is regular or irregular.

Solution: It is a regular because all of the sides are congruent and all of the angles are congruent.
C. Find the value of x in the quadrilateral.

Solution:
x+ 92+ 111+ 73 = 360
x+ 276 = 360
x = 84
Now let's test your knowledge :)
Try these problems on your own.
A. Tell whether the figure is a polygon.
1.

2.

B. Tell whether the polygon is regular or irregular.

C. Find the value of x in the figure.

A. Tell whether the figure is a polygon.
1.

Solution: Yes, it is a polygon because it's a closed figure that has 3 or more sides connecting at the vertices.
2.

Solution: No, it is not a polygon because it is not a closed figure.
B. Tell whether the polygon is regular or irregular.

Solution: It is a regular because all of the sides are congruent and all of the angles are congruent.
C. Find the value of x in the quadrilateral.

Solution:
x+ 92+ 111+ 73 = 360
x+ 276 = 360
x = 84
Now let's test your knowledge :)
Try these problems on your own.
A. Tell whether the figure is a polygon.
1.

2.

B. Tell whether the polygon is regular or irregular.

C. Find the value of x in the figure.

Monday, January 5, 2009
Introduction
This blog is under construction. It is designed as a place for my 3rd period Geometry students to display examples of how to use the information presented in chapter 6 of their coursework. There are 7 groups and each group is responsible for one of the 7 sections in chapter 6. Each post should be titled using the section number and title.
Subscribe to:
Posts (Atom)